Algorithm 10.2: Local Newton for optimization using the quadratic model. More...

Functions
function	newtonLocalQuadratic (in obj, in x0, in eps, in cg, in maxiter)
	Applies local Newton algorithm to solve 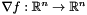$\nabla f(x)=0$ where $\nabla f:\mathbb{R}^n\to\mathbb{R}^n$ is the gradient of the objective function. More...

Detailed Description

Algorithm 10.2: Local Newton for optimization using the quadratic model.

Implementation of algorithm 10.2 of [1]

Function Documentation

function newtonLocalQuadratic	(	in	obj,
		in	x0,
		in	eps,
		in	cg,
		in	maxiter
	)

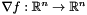Applies local Newton algorithm to solve $\nabla f(x)=0$ where $\nabla f:\mathbb{R}^n\to\mathbb{R}^n$ is the gradient of the objective function.

Note: Tested with run0508quadratic.m; Calls quadraticDirect; Calls conjugateGradient

Parameters

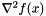
obj	the name of the Octave function defining $\nabla f(x)$ and the hessian $\nabla^2 f(x)$
x0	the starting point
eps	algorithm stops if $\\|F(x)\\| \leq \varepsilon$ .
cg	if 0, the quadratic model is solved using the direct method. If not zero, using the conjugate gradient algorithm.
maxiter	maximum number of iterations (Default: 100)

Returns: [solution,f]; solution: root of the function; f: value of F at the solution