|
English only
|
|

|
Information
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
David Simchi-Levi | |||||
|

|
||||
Gábor Tardos |
|||||
|

|
||||
Robert Weismantel |
|||||
|

|
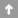
| Sunday 16 | Monday 17 | Tuesday 18 | Wednesday 19 | Thursday 20 | |
|---|---|---|---|---|---|
| 08h30 - 09h00 | Tardos | Simchi-Levi | Simchi-Levi | Tardos | |
| 09h00 - 09h30 | |||||
| 09h30 - 10h00 | |||||
| 10h00 - 10h30 | break | break | break | break | |
| 10h30 - 11h00 | Tardos | Tardos | Simchi-Levi | Simchi-Levi | |
| 11h00 - 11h30 | |||||
| 11h30 - 12h00 | |||||
|
12h00 - 17h00 |
Sport and discussions | ||||
| 17h00 - 17h30 | Weismantel | Atasoy | Bock | ||
| 17h30 - 18h00 | Boyacy | Buergisser | |||
| 18h00 - 18h30 | Welcome cocktail | Fuchsberger | Uldry | ||
| 18h30 - 19h00 | Niemeier | Wagner | |||
Sorry, the deadline for registration is over.
Current number of registered participants: 49
Presentations by PhD students will be organized.
Current number of submitted abstracts: 10
In the context of airline scheduling, integrated schedule design and fleet assignment model is studied in the literature with the purpose of increasing the revenue by determining the schedule simultaneously with fleet assignment. In this study we further integrate the demand modeling into the optimization problem where the spill effects are taken into account. Therefore the joint decision of pricing, fleet assignment and schedule design determines the actual number of passengers flying on each itinerary with the objective of maximizing the difference between revenue and operating costs. The demand modeling is done with linear, exponential, and piecewise linear functions where the explanatory variable is the fare of the itinerary.
The SBRP is a variant of the well-studied Vehicle Routing problem. Its objective is to minimize the number of vehicles used to carry a given set of children to school. The bus routes respect a uniform vehicle capacity and a bound on the maximum difference between the travel time of a child on the bus and a direct transport to school. Due to its inherited NP-hardness, we look for an approximate solution in theory and practice. A constant factor approximation algorithm on trees is presented. In practice, the SBRP can be solved using a column generation approach. We will report results on a real-life instance.
The Capacitated Multi-facility Weber Problem is concerned with locating m facilities in the plane, and allocating their capacities to n customers at minimum total cost, which is a non-convex optimization problem and difficult to solve. In this work we relax the capacity constraints and solve the uncapacitated Lagrangean subproblems every step of a subgradient algorithm. Their objectives have facility dependent distance functions, which is different than the usual multifacility Weber problem. We solve them by branch-and-price using column generation with concave minimization pricing.
We show that the Hedge Algorithm, a method that is widely used in Machine Learning, can be interpreted as a particular subgradient algorithm for minimizing a well-chosen convex function, namely as a Mirror Descent Scheme. Using this reformulation, we establish three modifications and extensions of the Hedge Algorithm that are better or at least as good as the standard method with respect to worst-case guarantees. Numerical experiments show that the modified and extended methods that we suggest perform consistently better than the standard Hedge Algorithm.
A dispatching support system for the station area of Berne, Switzerland is presented. The model assigns to each train a path based on a variety of alternative track routes, station platforms, speed profiles and arrival or departure times. While respecting safety regulations based on detailed blocking time theory, the optimal assignment is minimizing a weighted combination of delay propagation and number of broken connections. The model is iteratively adapted to the current state situation and solved in a rolling time horizon framework to cope with the continuously changing online situation.
The closest lattice vector problem is one of the central computational problems in the geometry of numbers. Here one is given a rational lattice, i.e. set set of integer combinations of a basis A of the vector space Q^n and a vector t of Q^n. The task is to compute a vector of the lattice that is closest to t w.r.t. a given norm. We will present the currently fastest known randomized (1+eps)-approximation algorithm for the closest vector problem in infinity norm. It is based on the following geometric problem: Cover the cube [-1+eps,1-eps]^n with parallelepipeds such that each parallelepiped, if scaled by 2, is contained in the cube [-1,1]^n. We present a covering scheme that allows to boost any constant factor approximation algorithm for the closest vector problem to a (1+eps) approximation algorithm, with a logarithmic dependence on 1/eps. This yields a (1+eps) approximation algorithm with a running time of 2^(O(n))(log 1/eps)^(O(n)), improving on the previously fastest algorithm by Bloemer and Naewe with a running time of (2+1/eps)^(O(n)).
We consider a delivery problem with a heterogeneous fleet of vehicles and several depots. The demands of the customers are typically larger than the capacity of the vehicles which means that most customers are visited several times. This is a split delivery vehicle routing problem with additional constraints, which can be modeled as an integer linear programming problem, that simultaneously assigns deliveries to the vehicles and builds vehicle routes. Experiments on real life instances are presented.
Managing shelf space is critical for retailers to attract customers and to optimize profit. The decisions relating to the products to be stocked among a large number of competing products and the amount of shelf space to allocate to those products is a question central to retailing. Consumer behaviour studies show that more a product is displayed on the shelf, more could be its sale. However the amount of space on the shelf is limited and a retailer also needs to stock competing products even if one of the products is a leader in terms of volume sales. This problem is complicated by the fact that the relationship between volume sales of the product and its number of facings is not linear.
A convex set with non-empty interior is maximal lattice-free if it is inclusion-maximal with respect to the property of not containing integer points in its interior. Maximal lattice-free convex sets are known to be polyhedra. Recently, it has been shown that, up to affine mappings preserving the integer lattice, the number of maximal lattice-free rational polyhedra of a given precision is finite. In this talk, I will explain the key steps of the proof and the underlying geometry.
Scheduling nuclear plants outages for refuelling and maintenance is a challenging optimization problem with a stochastic demand to satisfy by using different production units affected by random failure process. The present work deals with a very simplified model of this problem leading to a huge combinatorial problem with uncertain data. Using a probabilistic approach and conic approximations, we deal with a mixed integer second order conic program. We will present different conic approaches for its resolution.